(Sorry about the recent dearth of cp4space postings; I’ve been rather busy in real Euclidean 3-space, .)
Quite a large class of self-similar geometrical objects can be expressed as iterated function systems. For instance, the Sierpinski triangle is composed of three copies of itself, each scaled by a factor of ½ and translated by a cube root of unity. More elaborate self-similar sets can be generated by applying other combinations of affine transformations, such as the Barnsley fern:
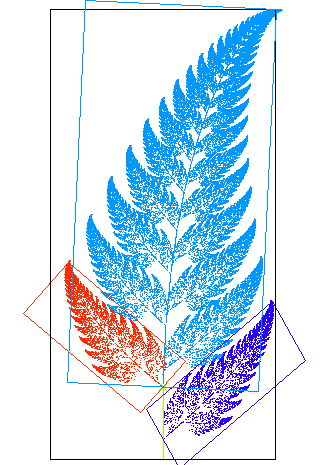
In most cases, the Hausdorff dimension is not an integer, in which case the object is referred to as a fractal. For instance, the aforementioned Sierpinski triangle has a dimension of log(3)/log(2). The Cantor ternary set, on the other hand, has a dimension of log(2)/log(3).
A rather paradoxical fractal is Antoine’s necklace. Firstly, you begin with a plain, bog-standard torus:
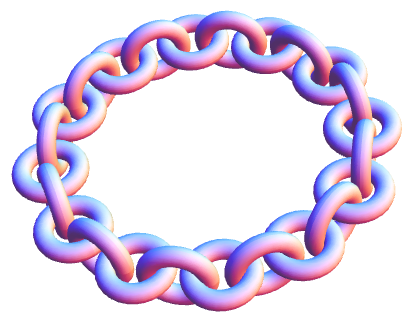
Then, place a circular chain of linked tori inside the torus, like so:
We can repeat this process inside each of these tori, and so on ad infinitum. Taking the intersection of the (nested) sets obtained at each stage, the result is homeomorphic to the Cantor set. However, the complement is not simply-connected, and therefore not homeomorphic to the complement of the usual embedding of the Cantor set in 3-space. More details are included here.
Antoine’s necklace can be used to construct another unusual object, Antoine’s horned sphere. This is obtained by taking a sphere, and extending a tentacle from its surface, which penetrates the initial torus in the construction of Antoine’s necklace. Inside the torus, the tentacle branches into more tentacles, which penetrate each of the sub-tori. This results in a tangled dendritic mess, which is homeomorphic to the 3-ball. However, quite alarmingly, the complement of the horned sphere is not homeomorphic to the complement of the 3-ball. Another such ‘exotic embedding’ of the 3-ball is Alexander’s horned sphere.


Pingback: Gears | Complex Projective 4-Space