After Zhang established the result that there are infinitely many pairs of primes separated by at most 70000000, there has been a massively collaborative effort to reduce this bound. The efforts are concentrated on developing methods to strengthen each of three variables in the original paper:
- The pair of real parameters (ω, δ), which were equal in the original paper, but later separated so that they can be optimised independently. Current efforts are to increase the value of ω in the hope of obtaining a smaller value of:
- The integer k0: This is a positive integer such that for each admissible set S of k0 integers, there are infinitely many translates S + n containing at least two primes. An admissible set is one where, for each prime p, there exists a residue class
disjoint from S. At the moment, there is an established upper bound of 26024 and a conditional result by Terry Tao et al claiming that
. Reducing the value of k0 leads to a smaller value of:
- The integer H, which is the diameter of the smallest admissible set of size k0. For each k0, it is possible to find upper bounds (using sieves to construct admissible sets) and lower bounds (obviously, k0 is a trivial lower bound, but certain inequalities such as Montgomery—Vaughan provide much tighter bounds). More details are here.
For the last few hours, the progress has rather resembled a game of tennis between Andrew Sutherland and xfxie, with the world record for H being repeatedly tossed between them. At the moment, Sutherland is winning, having reduced H down to 285278. You can get a slice of the action by viewing this comments thread, and perhaps chiming in with a smaller admissible set*.
As you can see, Sutherland has introduced a new optimisation together with a rather colourful piece of new terminology to describe it! This technique can sometimes lead to incremental (or should that be excremental?) reductions in the upper bound for H.
* Entries are to be given as a list with the filename admissXble_YYYYY_ZZZZZZ.txt, where X is a vowel used to specify the author (i for xfxie and a for Sutherland; you can choose any other vowel in your surname), YYYYY is the size of the set (preferably one of the established or conditionally proved values of k0), and ZZZZZZ is the diameter (an upper bound for H, assuming your value of k0 is correct). Examples are admissible_26024_286216.txt and admissable_10719_108514.txt.
We’re still waiting for confirmation that the value 10719 is valid; if so, the upper bound on H will drop from 285278 to 108514 — a significant improvement, but still considerably far from the desired target of 2. Here’s the timeline at the moment:
For continually-updated data, consult the polymath page.

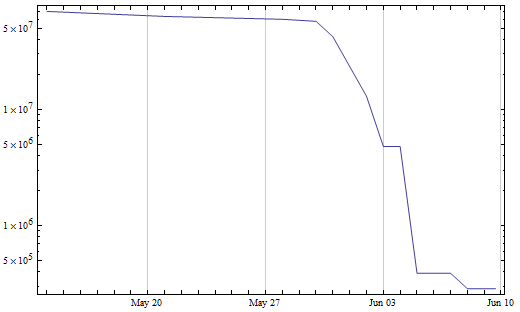
It’s great how a bit of competition can lead to such good results. We are 2 orders of magnitude closer to twin prime conjecture!
In related news, a new Olympic sport has been created.
248970 and still counting (backwards) …
Pingback: Assorted news | Complex Projective 4-Space
down to 6,966 confirmed via Maple by Terence Tao (k=0 via Hannes & H = 6,966 via Engelsma) – http://terrytao.wordpress.com/2013/06/23/the-distribution-of-primes-in-densely-divisible-moduli/#comment-236517