The second round of the British Mathematical Olympiad was marked today; results are available from Joseph Myers’ website. Congratulations to everyone who featured on the leaderboard (especially Linden Ralph, who secured first place). Moreover, best of luck to the recently-appointed EGMO team of Olivia Aaronson, Kasia Warburton, Eloise Thuey and Katya Richards.
31c/240 Caterpillar progress
In other news, Ivan Fomichev and Dave Greene have been working on building a 31c/240 spaceship in Conway’s Game of Life, using a similar principle to the (rather massive!) 17c/45 Caterpillar constructed by Gabriel Nivasch, David Bell and Jason Summers. Nivasch has written an excellently descriptive article about it, which I suggest reading before proceeding to familiarise yourself with the concepts and terminology.
Fortunately, the 31c/240 model is likely to be considerably smaller than its predecessor. In particular, the slow speed facilitates alternative mechanisms such as this one (henceforth referred to as Design II, to contrast with Design I used in the 17c/45 Caterpillar), where streams of gliders from the back catch up with the front to stabilise the behemoth:
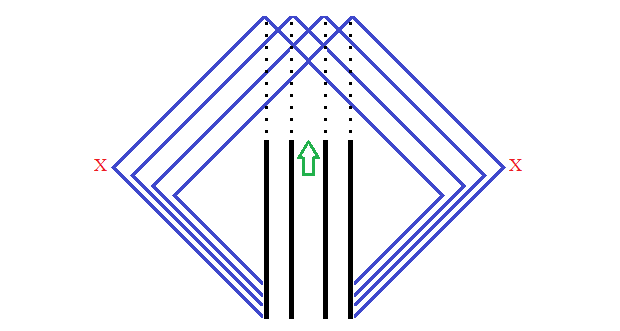
(This would have been impossible for the 17c/45 caterpillar, since the vertical component of the velocity of a glider is only c/4, which is slower than the spaceship’s speed of 17c/45; consequently, c/2 orthogonal spaceships were necessary instead.)
Dave Greene has built a complete front end based on Design II, and Ivan Fomichev has done the same for Design I (including constructing a helix). Note the red ‘x’ in the diagram, where forward gliders must be reflected inwards to stabilise the front; this presents an additional engineering problem. In fact, there are two suggested solutions:
-
Design IIa: using some static junk which will be pushed forwards at a speed of 31c/240 by the glider waves;
- Design IIb: colliding the glider waves with horizontal spaceships emitted from the spine.
It is universally acknowledged that IIb is more practical than IIa, so the current effort is to realise it. All of the individual components have been designed, so it is simply a matter of assembling them into a functional spaceship.
Exotic 7-spheres
Anyway, two smooth manifolds are homeomorphic if there exists a continuous bijection with continuous inverse, and diffeomorphic if there exists a differentiable bijection with differentiable inverse. Clearly, every diffeomorphism is a homeomorphism, but the converse is not true. That suggests the following question:
Do there exist smooth manifolds S and T which are homeomorphic but not diffeomorphic?
Amazingly, the answer is yes, as proved by John Milnor in the case where S is the 7-sphere (boundary of an 8-ball), in which case T is called an exotic 7-sphere. There are 27 of these beasts, which (together with the ordinary 7-sphere) form an order-28 abelian group (indeed, the cyclic group C28) under the operation of connect-sum.
It is an open problem as to whether there exist exotic 4-spheres. There are, however, exotic versions of , a phenomenon that occurs in no other dimension. Indeed, the situation is much scarier than the compact case of 7-spheres: there are uncountably many non-diffeomorphic smooth structures on four-dimensional space!

“While talking about this exact topic, let’s suddenly switch to another, completely unrelated, one” – every CP4space post, ever 😛
I also have an unrelated question: I was recently trying to find solutions for one particular problem, and the size of a solution was depended on size of a “base set”, which must be linearily independent over Q. For uncountable solution, we need an uncountable base set. It can be easily defined using axiom of choice (Zorn lemma or vector space basis existence, whichever you want), but I was wondering if such uncountable set is constructible, or, at least, provably existent without AC?
No, there exist models of ZF in which the reals do not have a Hamel basis as a Q-vector-space. Consequently, there is no constructive example.
But the axiom of choice is definitely true, so you’re allowed to use Zorn’s lemma.
I can’t really see why former implies latter. From what I understand, every Hamel basis must have size continuum (it might not be a case, as in ZF~C countable union of countables doesn’t have to be countable), and, if c>Aleph_1, there is still a possibility for us to make linearily independent set of size Aleph_1, which can’t be extended to basis, due to lack of AC.
Oh, you just want an uncountable Q-linearly-independent set of reals, rather than a Hamel basis? Sure, that’s possible. Take (for example) sum_(n=1 to infinity)[10^-floor(exp(xn))] for each real x > 1.