Poncelet’s porism: the Socratic dialogue

In the 1994 proceedings of the Mathematical Association of America, there is a truly wonderful article by Jonathan King. Entitled Three Problems in Search of a Measure, it provides three very different examples of problems that can be solved using elementary methods by considering the notion of a measure. The appeal of the article stems largely from the fact that the proofs are so clean and elegant, motivating measures and ergodic theory without having to rely on any advanced theorems.

One of the problems is the Arctic circle problem, which I first encountered in Geoff Smith’s Geometry Thoughts for the Day on 2011-06-11:

This problem is quite well known, and is related to something that has been discussed recently. A fishing hole in the ice forms a perfect circle of diameter d. A rich supply of arbitrarily thin planks of all sorts of length and widths is available. The hole must be covered with planks for Health ‘n Safety. Clearly this can be done with a finite collection of planks of total width d by placing them in parallel fashion.

Is it possible to cover the hole by using a finite collection of planks of total width less than d by using some kind of cunning criss-cross arrangement of the planks?

I’ll leave you to contemplate this; it’s very satisfying to solve.

Poncelet’s porism

Suppose we have an n-gon with both an incircle and a circumcircle. Poncelet’s porism is the theorem that we can then find infinitely many n-gons sharing the same incircle and circumcircle, by starting from any point on the circumcircle. If you prefer, Wolfram MathWorld has a very instructive animation of the statement of the porism:

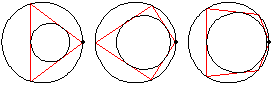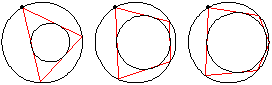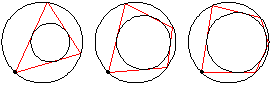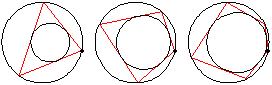
The usual ‘proof from the book’ of Poncelet’s porism involves the theory of elliptic curves. By comparison, the concepts in King’s proof are sufficiently elementary that I claim the proof could be understood by the Ancient Greeks. To emphasise this point, I shall present a slight reworking of King’s proof as a Socratic dialogue between Archimedes (who has hypothetically discovered the proof) and Eratosthenes. I have chosen Eratosthenes over the more usual candidates of:

• Meno’s slave (if you’re Plato);
• A Magdalene undegraduate reading Land Economy through the bottom of a Pimm’s glass (if you’re Piers Bursill-Hall);
• Tim Gowers’s son (if you’re Tim Gowers);

on the basis that I wanted a contemporary of Archimedes who would be able to understand the subtleties of the proof.

Quod erat demonstrandum. By the way, the result can be generalised to ellipses instead of circles by applying a projective transformation, although of course this would be beyond Ancient Greek mathematics so I decided against including it in the Socratic dialogue.