You may have wondered why the last few ciphers have been erratic. The answer is so that the 50th cipher will land exactly on Professor Imre Leader‘s 50th birthday, which was celebrated 14 hours ago. One of the main components of the celebration was this Othello cake I assembled from an existing cake, a layer of fruit compote, a sheet of icing, chocolate buttons of two varieties, and candles:
Mapping a square Othello board on a circular cake is easy, thanks to the famous Riemann mapping theorem. If Imre played three-dimensional Othello, then that would no longer be the case, as Liouville’s theorem asserts that the only conformal maps in three or more dimensions are Möbius transformations.
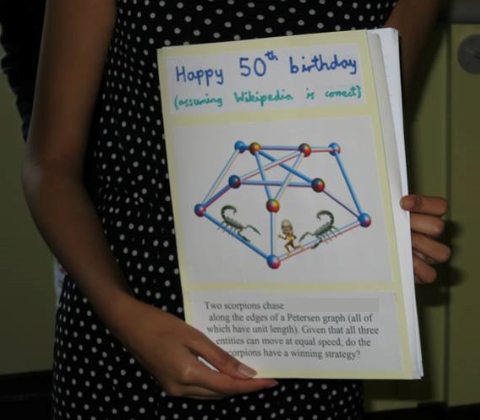
The birthday card was signed by fourteen A4 sheets of people, and featured the following pursuit-and-evasion problem inspired by an analogue on the edges of a tetrahedron. A name has been censored for legal reasons:
I believe that Gabriel Gendler was the first to solve this version of the problem. We also made a related board game by discretising space and time:
Anyway, here is the cipher, which you should decipher and follow as soon as possible:
Jnvg ba cbaf qryrpgngvbavf hagvy zvqavtug. Ybbx ng (2π/3, π/6). Gur cnffjbeq vf va ybjre-pnfr.
Good luck.



Unless I’m totally missing something, is this the first cipher where the deciphering is the easy part?
Indeed.
The answer doesn’t involve traveling to a physical location, does it?
That might be one possible way of doing it.
At two minutes to midnight on Imre Leader’s 50th birthday, yes. Do you have a time machine, per chance?
I wondered about that too, but wasn’t concerned since my time machine is working just fine. Can I email you with what I think needed to be done, and get the password if I’m correct?
You should be able to get the password without actually visiting the location, just by determining what would be there at that particular time.
Still thinking about this one from time to time. Would you be willing to say if the location is on or near to the Cambridge campus?